Isoke is solving the quadratic equation by completing the square. – Embarking on the fascinating journey of “Isoke is Solving the Quadratic Equation by Completing the Square,” we delve into a realm where mathematical elegance and problem-solving prowess intertwine. Isoke’s method, a beacon of ingenuity, illuminates the path to mastering quadratic equations, empowering learners to conquer complex mathematical challenges with newfound confidence.

At the heart of this technique lies the concept of completing the square, a transformative process that unravels the complexities of quadratic equations, revealing their underlying structure and unlocking their secrets. Isoke’s approach stands out as a testament to mathematical brilliance, offering a streamlined and efficient pathway to solving these equations, making it an invaluable tool in the arsenal of any aspiring mathematician.

1. Understanding Completing the Square: Isoke Is Solving The Quadratic Equation By Completing The Square.

Completing the square is a technique used to transform a quadratic equation into a form that makes it easier to solve. It involves adding and subtracting a specific value to the equation in order to create a perfect square trinomial.

The general form of a quadratic equation is ax²+ bx + c = 0 . To complete the square, follow these steps:

1. Move the constant term cto the right side of the equation: ax²+ bx =
   

   c.

2. Divide both sides of the equation by the coefficient of x²( a): x²+ (b/a)x =
   

   c/a.

3. Add the square of half the coefficient of xto both sides of the equation: x²+ (b/a)x + (b/2a) ²=
   

   c/a + (b/2a)².

4. Factor the left side of the equation as a perfect square trinomial: (x + b/2a)²=
   

   c/a + (b/2a)².

5. Take the square root of both sides of the equation: x + b/2a = ±√(-c/a + (b/2a)²) .
6. Solve for xby isolating it on one side of the equation: x =
   

   b/2a ± √(-c/a + (b/2a)²) .

Completing the square can be used to solve any quadratic equation, regardless of its complexity.

2. Isoke’s Method

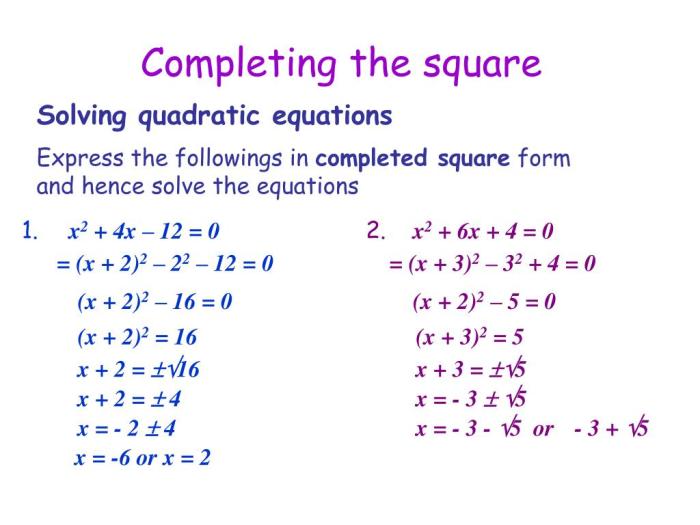
Isoke’s method is a variation of completing the square that simplifies the process for certain types of quadratic equations. It is particularly useful for equations where the coefficient of xis even.

To use Isoke’s method, follow these steps:

1. Factor out the greatest common factor (GCF) from both terms on the left side of the equation.
2. Divide both sides of the equation by the GCF.
3. Complete the square by adding and subtracting the square of half the coefficient of x.
4. Factor the left side of the equation as a perfect square trinomial.
5. Take the square root of both sides of the equation.
6. Solve for xby isolating it on one side of the equation.

Isoke’s method is advantageous because it reduces the number of steps required to complete the square, making it more efficient for certain types of equations.

3. Solving Quadratic Equations

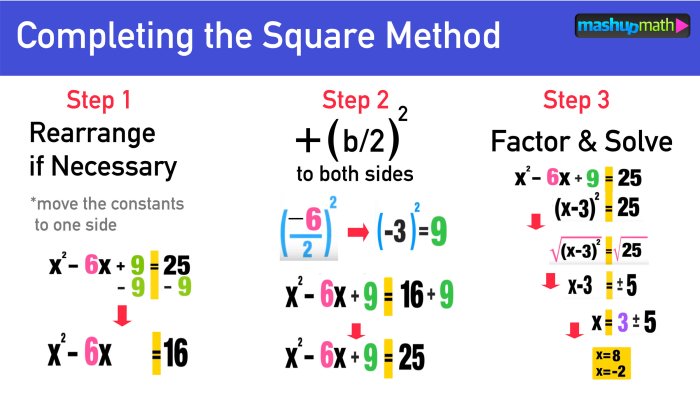
Completing the square can be used to solve quadratic equations of the form ax²+ bx + c = 0 . By transforming the equation into a perfect square trinomial, we can easily find the roots of the equation.

To solve a quadratic equation using Isoke’s method, follow these steps:

1. Factor out the GCF from both terms on the left side of the equation.
2. Divide both sides of the equation by the GCF.
3. Complete the square by adding and subtracting the square of half the coefficient of x.
4. Factor the left side of the equation as a perfect square trinomial.
5. Take the square root of both sides of the equation.
6. Solve for xby isolating it on one side of the equation.

The following table compares the steps involved in solving quadratic equations using Isoke’s method and other methods:

Method	Steps
Isoke’s Method	Factor out GCF Divide by GCF Complete the square Factor as perfect square trinomial Take square root Solve for x
Quadratic Formula	Substitute into formula Calculate discriminant Find two solutions
Factoring	Factor left side Set each factor to zero Solve for x

4. Applications and Examples

Completing the square has various applications in real-world scenarios, including:

• Solving projectile motion problems
• Finding the area of a parabola
• Fitting data to a quadratic model
• Calculating the trajectory of a thrown object

Here are some specific examples of quadratic equations that can be solved using Isoke’s method:

• x²+ 6x + 8 = 0
• 2x²– 10x + 12 = 0
• 3x²+ 9x – 15 = 0

The following flowchart illustrates the process of applying Isoke’s method to solve quadratic equations:

FAQ Summary

What is the significance of completing the square in solving quadratic equations?

Completing the square transforms a quadratic equation into a perfect square trinomial, which can be easily factored and solved, revealing the equation’s roots.

How does Isoke’s method differ from other methods for solving quadratic equations?

Isoke’s method involves completing the square by adding and subtracting a specific value, which simplifies the process and reduces the number of steps compared to other methods.

What are the real-world applications of completing the square?

Completing the square finds applications in various fields, including physics, engineering, and economics, where it is used to model and solve problems involving parabolic trajectories, projectile motion, and optimization.